Do Rational Numbers Include Decimals

Decimal Representation of Rational Numbers

A rational number is a number that tin can be written in the form p/q where p and q are integers, and q ≠ 0. The set of rational numbers is denoted by Q or \(\mathbb{Q}\). Examples:i/4, −two/5, 0.three (or) 3/10, −0.vii(or) −7/10, 0.151515... (or) fifteen/99. Rational numbers can exist represented as decimals. The different types of rational numbers are Integers like -1, 0, 5, etc., fractions like 2/5, i/iii, etc., terminating decimals like 0.12, 0.625, 1.325, etc., and non-terminating decimals with repeating patterns (after the decimal betoken) such equally 0.666..., 1.151515..., etc.

one.	Decimal Representation of Rational Numbers
ii.	Decimal Representation of Terminating Rational Number
iii.	Decimal Representation of Non-Terminating Rational Number
4.	Solved Examples on Decimal Representation of Rational Numbers
5.	Exercise Questions on Decimal Representation of Rational Numbers
6.	Frequently Asked Questions (FAQs)

Decimal Representation of Rational Numbers

The decimal representation of a rational number is converting a rational number into a decimal number that has the aforementioned mathematical value as the rational number. A rational number tin can exist represented as a decimal number with the assistance of the long division method. We separate the given rational number in the long division grade and the quotient which we go is the decimal representation of the rational number. A rational number can have 2 types of decimal representations (expansions):

Terminating
Non-terminating only repeating

Note: Any decimal representation that is non-terminating and not-recurring, will be an irrational number.

Let's try to understand what are terminating and non-terminating terms. While dividing a number by the long division method , if we go zippo as the remainder, the decimal expansion of such a number is called terminating.

Example: 1/2

Let usa see the long division of 1 by ii in the post-obit image:

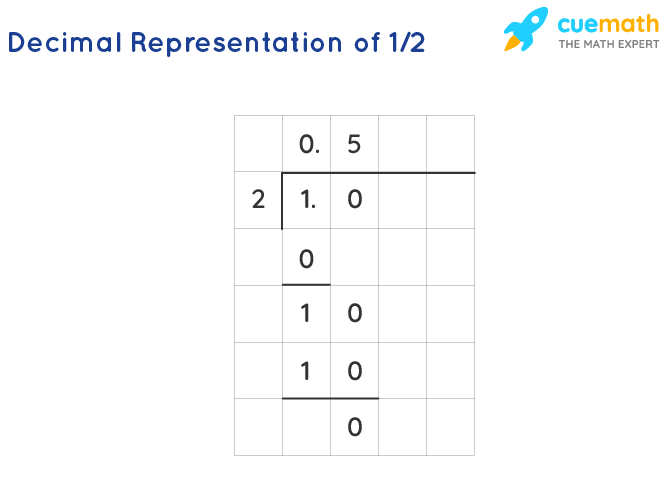
the rational number is terminating

1/2 = 0.5 is a terminating decimal

And while dividing a number, if the decimal expansion continues and the rest does not go aught, it is called not-terminating.

Case: 1/3

Permit usa see the long division of 1 by three in the following prototype:

Division of 1 by 3 is non terminating and recurring.

1/3 = 0.33333... is a recurring, non-terminating decimal. You can notice that the digits in the quotient keep repeating.

Decimal Representation of Terminating Rational Number

The terminating decimal expansion means that the decimal representation or expansion terminates later on a certain number of digits. A rational number is terminating if information technology can be expressed in the form: p/(2^north×five^m). The rational number whose denominator is a number that has no other factor than 2 or 5, will terminate the result sooner or later subsequently the decimal point. Consider the rational number 1/16.

Decimal Representation of 1 divided by 16 - Terminating

Hither, the decimal expansion of 1/xvi terminates later on iv digits. Here 16 in the denominator is 16 = 2^four. Notation that in terminating decimal expansion, y'all will notice that the prime factorization of the denominator has no other factors other than ii or 5.

Decimal Representation of Non-Terminating Decimal Number

The non-terminating merely repeating decimal expansion ways that although the decimal representation has an infinite number of digits, there is a repetitive pattern to it. The rational number whose denominator is having a gene other than 2 or five, volition not accept a terminating decimal number as the consequence.

For case:

Decimal Representation of 1 divided by 7 - Non-Terminating, Repeating

Note that in non-terminating only repeating decimal expansion, you will find that the prime factorization of the denominator has factors other than two or v.

Oft Asked Questions(FAQs)

Is a Decimal a Rational Number?

Any decimal number can be either a rational number or an irrational number, depending upon the number of digits and repetition of the digits. Whatever decimal number whose terms are terminating or non-terminating simply repeating then it is a rational number. Whereas if the terms are non-terminating and not-repeating, then information technology is an irrational number.

How Do Yous Know if a Decimal is Rational?

We can know a decimal number is rational or not by various methods. We can if a decimal number can be expressed in the class p/q and q ≠ 0, then it is a rational number. Case: 0.25 = 25/100 is a rational number. Or we can cheque the number of terms and repetition of the terms to know if it a rational number or non. For example, 0.33333... is a rational number.

What are the Characteristics of a Rational Number When Written every bit a Decimal?

When expressing a rational number in the decimal grade, it can be terminating or non-terminating but repeating and the digits can recur in a design. Example: 1/ii= 0.5 is a terminating decimal number. 1/three = 0.33333... is a not-terminating decimal number with the digit three, repeating.

If it is non-terminating and non-recurring, it is not a rational number. Case:π is an irrational number since it has a value that is non-terminating and not-recurring.

Is Every Decimal Number Represented every bit a Rational Number?

No, every decimal number tin non be represented as a rational number. Not-terminating and non-repeating digits to the right of the decimal signal cannot be expressed in the course p/q hence they are not rational numbers. Merely other than these terms, all terms can be represented as a rational number or in the form of p/q.

Is 3.14 a Rational Number?

A rational number is a number that can be written as a fraction, a/b where a and b are integers, or that has terminating or non-terminating but repeating terms. Hence, the number 3.14 is a rational number, since it has terminating terms after the decimal indicate. If information technology would have has further terms extending to infinity, it would accept been called an irrational number. An irrational number cannot exist expressed in the form of p/q It has countless not-repeating digits after the decimal signal. Examples: π = iii.141592..., √ii=ane.414213…

What Cannot be the Decimal Representation of a Rational Number?

A decimal number, that has space terms where terms are not repeating itself, then information technology can not be a decimal representation of a rational number. For a decimal number to exist the decimal representation of a rational number, information technology should take terminating, or not-terminating but repeating terms.

How Practice You Find the Decimal Expansion of a Rational Number?

The decimal expansion of a rational number tin easily be constitute out past using the long division or past just writing the rational number in the form of p/q besides known as a fraction class. To convert fractions to decimals, just divide the numerator past the denominator. As information technology is a rational number, we will get a effect that is either terminating or not-terminating merely repeating. If the denominator is of the form (2 ^{due north} ×v ^m ), then the decimal is sure to terminate, or otherwise, it would repeat with a recurring pattern.